By Frank Boldissar
As summer approaches, it’s once again time to think about heat and your aquarium. So hook up your chiller, and away you go; right? Maybe or maybe not, but in any event, it’s a good idea for every aquarist to have a basic understanding of the factors that affect the temperature his/her aquarium.
In Part 1 of this article, we go over the basics of heat transfer theory as applied to aquariums. In Part 2, we complete the theory and go over some practical aspects including some measurements to verify and augment the theory. As always, there is no substitute of personal observation of your aquarium to assess the health of your animals, especially in light of the inexact nature of heat transfer analysis.
Temperature
Temperature is one of the most important parameters of an aquarium. Fish and invertebrates
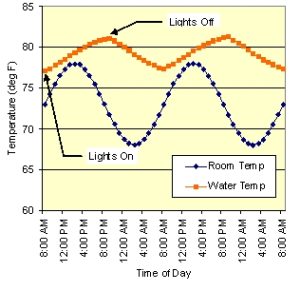
Figure 1: Simulated room temperature and water temperature 48 hour profiles for 100 gal tank with constant heat input from a pump and a 12 hour lamp cycle.
are cold blooded, and therefore, their body temperatures are entirely regulated by their environment. The temperature range requirements of an aquarium depend on the type of animals kept; it can fairly wide for some freshwater fish, or the range can be much narrower for coral species. Also, extremely rapid temperature changes, even within the recommended temperature range, can stress animals resulting in illness or death.
Unless your home has very precise thermal control, it is likely to experience daily thermal cycling. Over the course of 24 hours, the room temperature goes through a cycle of low temperature in the early morning to high just past mid-day and back to low again, and this temperature range is sometimes (much) larger than the tropical environment. Fig. 1 shows typical room temperature and aquarium temperature profiles for a 100 gal tank over a 48 hour period. The tank’s average temperature of 79F is 6 F deg hotter than the average room temperature due to the heat input from the lamps and pumps. However, the tank’s peak-to-peak variation is only ±2 F compared to ±5 F for the room, mainly because it takes quite a bit of heat to change the temperature of 100 gal of water. A smaller tank would have a variation closer to the room temperature variation.
Heat and Temperature
What is heat and how is it related to temperature? Heat, symbolized by Q, is a form of kinetic thermal energy that flows between objects, or within an object, due to a difference in temperature. Heat flows into your tank from the pump, lights, and the heater, of course. Heat flows out of the tank through evaporation, and the chiller, if you are so lucky as to own one. The room temperature can either heat or cool the tank, depending on whether the tank is hotter or cooler than the room. This relationship is shown schematically in Fig. 2.
Heat is usually measured in Joules, calories, or British Thermal Units (BTUs). One BTU is the heat required to raise 1 lb of water by 1 F deg, and one calorie is defined to be the amount of heat required to raise temperature of 1 gram of water 1 C deg. Note that 1 “calorie” of food energy = 1000 calories (or 1 kcal) for you fatties. This paper will use mostly English units, but conversions between English and SI units are given in Appendix A.
Specific Heat
A material property called Specific Heat, symbolized by c, relates heat and temperature. When we add one BTU to an object, its temperature goes up. The increase in temperature depends on the object’s mass and its specific heat. A material’s specific heat is defined as the amount of heat required to change a unit mass of the material by one degree in temperature. The specific heat of pure water is 1.00 BTU/lb/F, by definition. Seawater is slightly lower at about 0.93 due to its higher density. Multiplying by the density of water, r, and con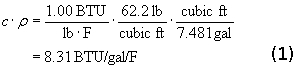 verting to gallons (US) gives us the amount of heat needed to increase the temperature of a gallon of water by 1 F deg:
verting to gallons (US) gives us the amount of heat needed to increase the temperature of a gallon of water by 1 F deg:
Fig. 3 shows the number of BTUs required to raise the tank temperature by 1 F deg. It generally takes 100’s of BTUs to raise the temperature even a small amount, and we need 1000’s of BTUs to change to temperature by 10 F. As you can see, large amounts of heat must be moving into and out of our tanks.
Heat Transfer
Heat transfer, or heat flow rate, is the amount of heat per unit time (BTU/hr) that is transferred to and from the water. Heat flowing into the tank is positive, heat flowing out is negative. If the aquarium has a net heat gain, the temperature increases; heat loss cools the tank. Once we know the heat flow rate, we can use the specific heat to find the change in temperature of the water. Hea
t flow can also be measured in watts (W) with 1.0 BTU/hr = 0.293 W. Note that a heater that produces 100 W of heat generates about 340 BTU/hr. Chillers are also rated in BTU/hr; a quarter horsepower unit will reportedly remove about 3,000 BTU/hr.
Since heat is a form of energy and must be conserved, so the total amount of heat flowing into the aquarium must equal the heat flowing out plus the change in internal energy of the water (also known as the First Law of Thermodynamics). The internal energy of the water is increased by heating up. In equation form:
Using the specific heat of water, we can substitute the change in energy with change in water temperature:

At first, equation (3) seems to tell us that the more heat we add on the left side, the hotter and hotter the water gets on the right side. Recall, however, that heat flows between two objects with a difference in temperature. Therefore, as the water gets hotter, say from a hot water pump for example, the difference in temperature between the water and pump actually gets smaller and so does the heat flow. Eventually the net heat flow goes to zero at equilibrium when the water temperature equals the pump temperature. Normally the tank water doesn’t get as hot as the pump. That’s because the net heat flow goes to zero. For aquariums, this usually means that the heat from the lights and pump is balanced with cooling by the ambient room temperature.
This principle can be illustrated by a simplified concept of heat flow called Newton’s Law of Cooling. Newton’s Law of Cooling states that the heat flow between an object and its surrounding environment can be characterized by a heat transfer coefficient, h, such that:
Although not always accurate, the concept of heat transfer coefficient will be useful in the rest of our discussion, because it describes how readily heat flows from one object to another.
For the case where the net heat flow is zero, Newton’s Law of Cooling and the first law of thermodynamics, equations (3) and (4), can be equated and solved to show how the water temperature changes with time. Given that a tank begins with an initial water temperature of Tinitial , we find that the water temperature varies exponentially with time as:
Equation (5) is plotted in Fig 4 for 10 gal and 100 gal glass tanks and a 10 gal acrylic tank in a hot room. We can see that, in all cases, the water temperature eventually becomes the temperature of the surrounding environment. How quickly that happens depends on the water volume, heat transfer coefficient and the heat transfer surface area. The water heats slower for larger volumes and smaller transfer coefficients. Note that a larger tank has more volume, but also more surface area. A 10 gal acrylic tank with a smaller heat transfer coefficient heats even more slowly than a 100 gal glass tank.
When we add additional constant heat flow into the tank, the temperature still has an exponential dependence, except that the final temperature is higher than the room temperature. Fig 5 shows the cooling of the 10 gal glass tank with and without a net heat flow into the tank. The extra 50 BTUs could come from lights or pumps, but the effect is that the tank runs 5 deg warmer than the room.
Next, let’s look at the four basic mechanisms of heat transfer into and out of our aquariums:
- Conduction – heat conducted through the walls of the tank
- Convection – heat transferred to/from moving water inside the tank or air on the outside
- Radiation – heat radiated or absorbed through radiation (primarily at infrared wavelengths)
- Evaporation – heat lost from water by evaporation
In each of these heat transfer modes, we strive to express the heat flow, q, in a form of Newton’s Law with an effective heat transfer coefficient to simplify the calculations.
Conductive Heat Transfer
Most people are familiar with conductive heat transfer. Conduction takes place when a temperature difference exists in a material. For an aquarium, water is heated or cooled by conductive heat transfer across the walls of the tank. The equation used to express heat transfer by conduction is known as Fourier’s Law and is given by:
And the effective conduction heat transfer coefficient is given by:
Note that heat flows more readily for larger surface areas, larger temperature differences, and thinner walls. The thermal conductivity, k, depends on the type of material; the higher the conductivity, the easier heat flows through it. The thermal conductivity of materials commonly found in aquarium systems is listed in Table 1. We can see from the table that glass aquariums gain/lose heat five times more quickly than acrylic tanks of the same thickness. Thus, acrylic tanks are generally more stable with sudden changes in room temperature that you might experience during a short term heat wave, as seen in Fig 4.
| Material | Thermal conductivity (BTU/hr/F/ft) |
|---|---|
| Aluminum | 144.0 |
| Glass | 0.61 |
| Water | 0.34 |
| Acrylic | 0.12 |
| Hardwoods | 0.09 |
| Styrofoam | 0.02 |
| Air | 0.014 |
Convective Heat Transfer
Convective heat transfer is a little less intuitive. Convection is heat energy transferred between a surface and a moving fluid (typically water or air) when the surface and fluid are at different temperatures. Convective heat transfer may take the form of either forced or natural convection. Forced convection occurs when the fluid is moved by an external force, such as a pump, or fan. Natural convection is caused by temperature variations in the fluid. For example, when air is heated, its density changes, causing the air to rise and be replaced by cooler air that also will heat and rise.
In an aquarium, convection accounts for heat transfer between the water and the inner tank walls and heat transfer between the outer walls and the room air. Convection also occurs between water and the pumps, heaters, and chillers, and also between lamps and the air above the tank.
Newton’s Law of Cooling is used to express heat transfer by convection:
The convection heat transfer coefficient, hconv, is generally too complex to calculate for most fish-keepers and thermal engineers. Therefore, typical empirically-derived convection coefficients are often used. For air and water cooling, hconv is generally within the ranges given in Table 2, with the low end representing natural convection and the high end forced convection. As you can see, water is 50 – 100 times more effective in transferring heat than air, and forced convection is about 10 – 20 times more effective than natural convection. For comparison to thermal conduction, a quarter-inch thick piece of glass has an effective transfer coefficient of 29.3 BTU/hr/F/sq ft.
| Material | Convection coefficient (BTU/hr/F/sq ft) |
|---|---|
| Air | 2 – 20 |
| Water | 100 – 2,000 |
Radiation Heat Transfer
Heat can also be transferred through thermal radiation. All objects emit electromagnetic waves in the wavelength range of 0.1 to 100 microns, based on their temperature. The heat radiates across space to cooler objects that can absorb it. This is how the sun warms the earth and how a fireplace heats a room. In an aquarium, the main radiation heat transfer occurs at infrared (IR) wavelengths for two cases: (1) transfer between the lamps and the water and (2) transfer between the aquarium outer walls and the room, with (1) being the dominant transfer mode.
Radiation heat transfer between two objects is rather complex involving the temperatures of the objects, the IR properties of the two objects, and the geometry of the objects. The heat radiated from a hot object at temperature T1 to a cool object at T2 is given by the Stefan-Boltzmann Law:
When DT = T1 – T2 << mean temperature (in Rankin), we can approximate the Stephan-Boltzmann Law with an effective radiation heat transfer coefficient, hrad, like we did with conduction:
For aquarium environments, equation (10) is valid for T1 < 200 F or so, which will suffice for radiation from the aquarium walls and fluorescent lamps, but may not be accurate for those hot metal halides systems.
The transfer function, F1-2, is a unit-less quantity that contains the material emittance and a “view factor” that accounts for the geometry of the two surfaces. The emittance, , varies from 0 to 1 and is a measure of the efficiency of the emitter. The emittance for materials commonly found in aquarium systems is listed Table 3. Higher emittance means higher heat radiation transfer.
| Material | Emittance |
|---|---|
| Aluminum, polished | 0.039 – 0.057 |
| Black paint | 0.80 |
| White paint | 0.87 |
| Plastics | 0.91 |
| Glass | 0.92 |
| Water | 0.95 – 0.963 |
Other than polished aluminum, most materials in Table 3 are good emitters and also good absorbers of IR heat (with white paint an exception – its absorptance is about 0.3).
For the case of the aquarium walls radiating to a much larger room, the transfer function is given by F1-2 = e1. Plugging in the numbers at aquarium temperatures gives a radiation heat transfer coefficient that is about the same as the natural convection in air – i.e. not tremendously strong.
The situation is much more complex for lamps. Most bulbs are installed in a housing with a reflector and a glass or plastic cover. Much of the heat generated by bulbs is reflected by the polished reflectors and absorbed by the cover. The cover then re-radiates the heat to the water. Some of the heat from the bulbs is removed by fan-driven convection cooling and also conducted to the housing that is in turn cooled by convection. Ultimately, the primary mode of radiation heat transfer occurs between the lamp covers and the aquarium water. For power compact fluorescent bulbs the lamp cover might get to 100 F or so, depending on the system, ventilation, etc. Metal halide covers might get as hot as 200 F or more.
The best way to evaluate the amount of heat entering your tank is by observing the temperature with and without lights on (more on this in Part 2), but we can get an idea of the magnitude by using equation (9) and making a few assumptions. For instance, if we assume that we have power compact lamps that uniformly heat a plastic cover that spans the entire tank top to a temperature of 120 F, we get about 45 BTU/hr/sq ft of surface area (tank top). That amounts to 63 BTU/hr for a 10 gal tank and 340 BTU/hr for a 100 gal tank.
Evaporative Heat Loss
Evaporation is the poor man’s chiller. I say this because fans are a lot cheaper than chillers. But while evaporation can remove hundred’s of BTUs per hour, chillers can do thousands. Evaporation is most effective in areas with low humidity and when assisted with a fan.
An easy way to compute the amount of evaporative cooling is to convert the amount of water that evaporates from your tank to BTUs/hr. The energy required to change from the liquid state to vapor is called the latent heat of vaporization, he. The latent heat of water is 8050 BTU/gal. To compute the amount of heat loss your aquarium is experiencing from evaporation, simply multiply the amount of water replaced per week by 8050 and divide by the number of hours in a week (168 hrs) = 48 BTU/hr for each gal replaced per week.
In lieu of knowing the amount of water evaporated, the heat loss can be computed from an empirical expression that is a function of the open surface area, the relative humidity, and the velocity of the air directly above the tank surface:
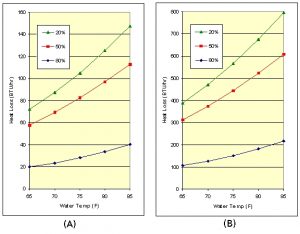
The humidity ratios are obtained from psychrometric charts such as the one in the Reference [3]. Although equation (10) is a bit complicated, it is useful for showing the sensitivity of evaporative cooling to humidity and air velocity. In general, humidity is the biggest factor, mainly because high air velocities are not possible with the smaller fans available for aquariums. Fig 6 shows the moderate amount of cooling as a function of relative humidity of 20%, 50% and 80% assuming zero air velocity. Fans will improve the evaporation rate, but more importantly, they also circulate the air over the tank so it doesn’t become saturated with humidity.
Figure 6: Evaporative cooling for a (A) 10 gal tank and a (B) 100 gal tank for various humidity levels and zero air velocity.
Conclusion of Part 1
We’ve covered the basics of aquarium heat transfer and the relative importance of the different transfer mechanisms. In Part 2, we will use the concept of thermal resistance to combine the various heat transfer coefficients to compute the complete heat flow through the aquarium and the resultant temperatures. We will also hopefully add some measurements to verify and augment the theory. As I mentioned at the beginning of this article, there is no substitute of personal observation of your aquarium to assess the health of your animals.
References
- J. Lienhard IV and J. Lienhard V, A Heat Transfer Textbook, Philogiston Press, Cambridge, MA, Web Edition 2008, http://web.mit.edu/lienhard/www/ahtt.html.
- The Engineering ToolBox, copywrite 2005, http://www.engineeringtoolbox.com/
- Coolerado Corp., Web Address, http://www.coolerado.com/tech-info/psychometric-charts/
| Parameter | English Units | Metric Units |
|---|---|---|
| Length | 1 ft | 0.3048 m |
| Area | 1 sq ft | 0.0929 sq m |
| Mass | 1 lb | 0.4536 kg |
| Volume | 1 cubic ft1 gal (US) = 0.134 cubic ft | 0.02832 cubic m1 liter = 0.001 cubic m |
| Density | 1 lb / cubic ft | 16.018 kg / cubic m |
| Energy | 1 BTU | 252 cal1055 Joules |
| Temperature | 1 F degT(F) = [T(C)](9/5) + 32 T(F) = [T(K) – 273](9/5) + 32T(R) = T(F) + 460 | 0.555 C degT(C) = 5/9[T(F) – 32] T(K) = 5/9[T(F) – 32] + 273 |
| Heat flow rate | 1 BTU / hr | 252 cal / hr0.293 W |
| Heat transfer coefficient | 1 BTU / (hr F sq ft) | 5.68 W / (K sq m) |
| Thermal conductivity | 1 BTU / (ft hr F) | 1.731 W / (m K) |
| Thermal resistance | 1 (hr F)/BTU | 1.8958 K / W |


















0 Comments